Physics, Chemistry, Biology
Invariance of quantum commutators through universal complex transformation
Prakash Barde NILESH 1, Pramodrao Bardapurkar PRANAV 2*
1Department of Physics, BadrinarayanBarwaleMahavidyalaya, Jalna, Maharashtra, India,
2* Department of Physics, S. N. Arts, D.J. Malpani Commerce & B. N. Sarda Science College, Sangamner, Maharashtra, India
E-mail(s): 1 nilesh_barde123@rediffmail.com; 2* ppbardapurkar@rediffmail.com
* Corresponding author phone: +919420808535
Received: March 16, 2018 / Accepted: June 05, 2018 / Published: June 30, 2018
Abstract
Commutators quantify the degree to an extent; two observables can be measured simultaneously. Quantum commutators are related by Universal Complex Transformations (UCT) which exhibit new symmetry of nature. Quantum parameters are concomitant to a wave function associated with a classical particle. It is imperative to know that few quantum parameters are invariant under UCT. Present article has a thrust to derive new commutation relations using UCT for various parameters and to exemplify the respective nature of invariance. In the present article, various quantum commutator relations are derived using UCT and the partial invariant nature of quantum commutators has been discoursed. The non-invariance of total angular momentum and Pauli spin operators is also evidenced. Few of the new derived commutation relations exhibit invariance in nature. Further, the effect of UCT on certain type of commutators, particularly the angular momentum and Pauli matrices is also discussed here.
Keywords
Universal Complex Transformation; Commutator; Invariance; Pauli matrices
Introduction
While exploring quantum mechanics to a variety of situations, physicists embrace an imaginary number i = Ö-1; utilized in constructing non-commutative relationships for various quantum parameters. Many quantum Physics models like Born-Jordan, Poisson bracket of Dirac, Schrodinger equation, Feynman’s path integral concept etc., employ this number “i”.
It seems that ‘i’ is indispensable in the formulation of quantum mechanics. Imaginary and complex numbers are much different than that of natural numbers and use of these numbers are necessary in formulating the theory of quantum mechanics[[1],[2],[3]].
In classical mechanics, various properties
of a system such as velocity, momentum, position, acceleration, force etc. may
be observed or calculated simultaneously. However, in case of quantum
mechanics, if properties of a particle are to be calculated, then the
measurement process is linked to an operator acting on the wave function
associated with the particle and this operator must be a pure eigen function.
For example, when two properties of a particle are to be determined
simultaneously, then particle must be in an eigen state of operators associated
with those two properties. It is very difficult to visualize this situation
theoretically. Consider, two operators; A
and B acting on any wave function “
”;
then the compact notation of operators will be [AB] = AB - BA, which is termed as commutator;
that commutes to zero or to the same value [[4],[5]].
Classically, operators are said to commute if AB - BA®0. If commutation of position x and momentum p is taken in account then classically; its commutation will be [x, p]®0, however, quantum mechanically, considering the uncertainty principle, [x, p]®ћ, where ћ= h/2p, that is Eq. (1):
(1)
Where: h - is Planck’s constant and i = Ö-1 is any imaginary. This commutation bracket helps to formulate quantum mechanics. It yields interesting physical results describing the quantum particles associated to the wave-functions. This is the generalized form of Poisson’s bracket in classical mechanics, which makes the transition from classical to quantum mechanics with the help of notions like Hamiltonian, Lagrangian and Newtonian formulations etc [[6]].
There are various physical parameters in classical mechanics like angular momentum, total angular momentum which show change in result if operated by different mathematical operators. However, quantum mechanically, these parameters may not show any change in the final result, though the operators are different. This underlines the thrust of this paper which aims to show the invariance nature of such parameters with reference to UCT. We have shown new commutation relations for certain transformation and approximations. The new symmetry exhibited by these results may be used to formulate certain quantum mechanical equations as Schrodinger wave equation, equations for particles trapped in different potential wells etc. The results obtained in the present work reveal that physical world must be invariant under UCT.
The obtained results may further be used in formulating quantum mechanics based on developed commutator brackets by introducing an imaginary number i®-i. To this end, it is worth to mention that though the present work paper does not directly formulate any new equation in quantum mechanics, it certainly explores at least a new direction that may appear worthwhile.
Material and Method
In this short article, an invariance of commutators is discussed. If an operator commutes with Hamiltonian of the system, then the dynamical variable corresponding to that operator is said to be conserved [[7]]. The commutation relations for angular momentum, total angular momentum (TAM) and ladder operators are derived here, along with their respective transformations. Derivation of these relations suggests their invariance nature under the complex transformations. In universal complex transformation (UCT), an imaginary term i is transformed as i®i. It has been assumed that the UCT must be symmetric in nature and the physical world is invariant under this UCT [[8],[9],[10]].
The steps involved in determination of commutation relations with and without UCT are highlighted in following flow charts, Figure 1.
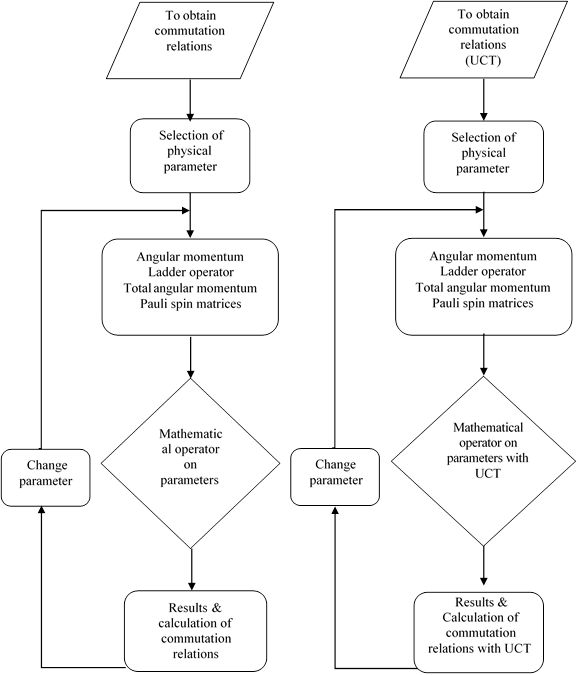
Figure 1. Flow charts elaborating steps involved in obtaining commutation relations through complex calculations
Results and discussion
Generalized commutation relations
Angular momentum: Angular momentum L is known for a vector operator whose three components do not commute with each other. It is represented as, L = r´p where, r and p represent the position vector and linear momentum respectively.
Now in Eq. (2), we have:
r = ix + jy + kz, p = ipx + jpy + kpz, L = iLx + jLy + kLz (2)
Where: x, y, z - cartesian coordinates for position vector r; px, py, pz - cartesian components for momentum p while Lx, Ly, Lz - cartesian components of angular momentum L.
Considering the momentum operator, p
with its cartesian components given by
, the angular momentum can be
derived as Eq. (3):
(3)
Where: 
, 
and
are the partial differentiation with
reference to position vectors x, y, and z respectively. Relations for angular
momentum, obtained without employing complex transformation are derived and the
results are summarized in Table 1. Here, L2 is the second order
partial differentiation, L+ = Lx + iLy
and L- = Lx - iLy are ladder operators
for angular momentum.
Table 1. Relations obtained without using complex transformation
|
S. N. |
Relations |
|
1 |
[Lx,Ly] = iћLz |
|
2 |
[Ly,Lz] = iћLx |
|
3 |
[Lz,Lx] = iћLy |
|
4 |
[Lx,px] = [Ly,py] = [Lz,pz] = 0 |
|
5 |
[L2,Lx] = [L2,Ly] = [L2,Lz] = 0 |
|
6 |
[Lz,L±] = ± ћL±; [L+,L-] = 2ћLz |
By introducing operators on commutator bracket, we obtained results through complex calculations. The results shown in Table 1 interpret that the commutation relations remains same even if mathematical operators are used on angular momentum, which is equivalent to the results obtained classically. These results are not efficient in deriving new form of equations in quantum mechanics.
Total angular momentum (TAM): The TAM, represented as J, is the sum of orbital and spin angular momentum i.e. J = L + S. Using momentum operator relations, we have Eq. (4):
(4)
Thus, various relations for TAM can be obtained and are presented in Table 2.
Table 2. Relations obtained without using complex transformation
|
S. N. |
Relations |
|
1 |
[Jx, Jy] = iћJz |
|
2 |
[Jy, Jz] = iћJx |
|
3 |
[Jz, Jx] = iћJy |
|
4 |
[J2, Jx] = [J2,Jy] = [J2,Jz]= 0 |
Results in table 2 show classical values of total angular momentum which is not useful in solving complex equations in quantum mechanics where there is a need to evaluate certain parameters using the commutator bracket which generalizes the Poisson bracket of classical mechanics.
Ladder operators: These operators can be represented in terms of TAM as Eq. (5),
J+ = Jx + iJy and J- = Jx-iJy (5)
Thus, the commutation relations derived for ladder operators are listed in Table (3).
Table 3. Commutation relations for ladder operators
|
S. N. |
Relations |
|
1 |
[Jz,J+] =ћJ+ |
|
2 |
[Jz,J-] = -ћJ- |
|
3 |
[Jz,J-] = -ћJ- |
|
4 |
[J+,J-] = 2ћJz |
|
5 |
[J2,J+] = [J2,J-] = [J2,J±] |
The ladder operators are related to total angular momentum and which when operated mathematically, show invariant nature. This demonstrates that if an operator does not commute, then the variable corresponding to the operator is said to be non-conserved.
The equations obtained in Tables (1) to (3) are the generalized form of non-commutative relationship between the momentums and positions. The formulation of these commutation relations emphasize importance of the imaginary number i, which eliminates the obscurity in the development of complex nature of quantum mechanics. With this context, we have adopted a differential commutator bracket involving UCT to formulate the new relations.
Commutation relations using UCT
The various expressions were derived by considering the complex transformation i®-iand the results obtained for angular momentum, TAM and ladder operator were compared with non-UCT results. This comparison is presented in table (4). Due to the space limitation of an article, authors of this paper feel not to elaborate detail description of the relations derived, hence only the final results are tabulated.
Table 4. Relations derived considering the complex transformation 
|
S. N. |
With UCT |
Without UCT |
|
1 |
[Lx,x] = 0 |
[Lx,x] = 0 |
|
2 |
[Lx,y] = -iћz |
[Lx,y] = iћz |
|
3 |
[Lx,z] = iћy |
[Lx,z] = -iћy |
|
4 |
[Ly,z] = -iћx |
[Ly,z] = iћx |
|
5 |
[Lz,x] = -iћy |
[Lz,x] = iћy |
|
6 |
[Lx,pz] = iћpy |
[Lx,pz] = -iћpy |
|
7 |
[Lx,Ly] = -iћLz |
[Lx,Ly] = iћLz |
|
8 |
[Ly,Lz] = -iћLx |
|
|
9 |
[Lz,Lx] = -iћLy |
[Lz,Lx] = iћLy |
|
10 |
[L2,Lx] = [L2,Ly] = [L2,Lz] = 0 |
[L2,Lx] = [L2,Ly] = [L2,Lz] = 0 |
|
11 |
[Lz,L+] = ћL+ |
[Lz,L+] = ћL+ |
|
12 |
[Lz,L-] = ћL- |
[Lz,L-] = -ћL- |
|
13 |
[Lz,L±] = ±ћL± |
[Lz,L±] = ±ћL± |
|
14 |
[L+,L-] = 2ћLz |
[L+,L-] = 2ћLz |
Most of the results in the above table, specifically related to an angular momentum and position, do not show invariance nature while that of ladder operators exhibit the invariance. This infers that UCT does not have any impact on total angular momentum in relation with that of ladder operator, but it certainly affects momentum and position coordinates. These results obtained by solving the commutation relations using UCT helps to understand the complex nature of quantum mechanics. When an operators commutes, the variables corresponding to the operator is said to be conserved. The results obtained from the above results implies that it is possible to adopt a differential commutator bracket involving angular momentum, ladder operator and total angular momentum using UCT to formulate new equations in quantum mechanics.
Calculation of Pauli’s spin operators with UCT
Classically, it is assumed that any two objects can be distinguished from one another by marking one of them. But, at the atomic scale, molecules cannot be marked and their in-distinguish ability affects the properties of identical particles. These particles are regarded as the particles which when interchanged in the system, will not make any change in it. These particles can be distinguished from each other only when their respective wave packets do not overlap. To identify them individually, the concept of spin is introduced in quantum mechanics by explaining it in terms of operators called as spin operators. The spin matrices are assumed to be operators noted as sx, sy, sz. Since, these matrices behave similar to angular momentum operators, they also satisfy the commutation relations. The matrices in terms of auxiliary operators may be written as,
These are known as Pauli matrices associated with the components of spin angular momentum. Here, s2 = sx2 + sy2 + sz2 is Pauli matrix of second order in terms of sx, sy and sz.
Table 5. Commutation relations with and without UCT
|
S. N. |
With UCT |
Without UCT |
|
1 |
s2 = (sx)2 + (sy)2 + (sz)2 = 3 |
s2 = (sx)2 + (sy)2 + (sz)2 = 3 |
|
2 |
[sx,sy] = -2isz |
[sx,sy] = 2isz |
|
3 |
[sy,sz] = -2isx |
[sy,sz] = 2isx |
|
4 |
[sz,sx] = -2isy |
[sz,sx] = 2isy |
|
5 |
[s2, sx] = [s2, sy] = [s2, sz] = 0 |
[s2, sx] = [s2, sy] = [s2, sz] = 0 |
The commutation relations with and without UCT are derived and summarized in the table (5).These results show that use of UCT for all the commutation relations between spin operators does not show invariance nature. The only the relation that sustains is that fors2and individual spin operator. It is well known that spin is a property of elementary particles like protons; electrons etc. and is referred as a vector quantity. It is the classical analogue of angular momentum of a rotating body. The value of spin lies between +½ and -½ associated with Pauli operators in a fixed direction. The results obtained in the present work show that the application of UCT changes the commutation relation for spin operators which is case sensitive for + and - signs, but does not have any effect in case of s2.
Conclusions
This article presents new commutation relations that have been derived using Universal Complex Transformation (UCT). Commutation relations between angular momentum and different position coordinate; linear momentum and angular momentum itself does not show any invariance, whereas the relations between angular momentum and respective position coordinate exhibit such invariance. The commutation relations between angular momentum and the ladder operators found to reveal the invariance. The relations between different Pauli matrices do not show invariance while that between s2 and individual Pauli matrices reveal the invariance. With these observations, it can be stated that, UCT affects a certain type of commutators; particularly the angular momentum as well as Pauli matrices. Thus, partial invariance of commutator relations using UCT shows that the angular momentum cannot be changed. In this article, new commutation relations have been corroborated using UCT. It is fascinating to know that few of the relations of quantum parameters show invariant nature under UCT. It is also observed in the present studies that UCT is a factual law of nature in which few commutation relations are satisfied. If an operator commutes with the other, then the variable corresponding to the operator is said to be conserved. This is gratified by the results obtained in this article using UCT. Thus, UCT has a vital role in understanding quantum mechanics.
In quantum mechanics, particles are described by relativistic and non-relativistic wave equations. Some of the results obtained from the present study are useful in applying UCT for deriving Schrodinger equations and obtaining its solution in specified boundary conditions which may yield slightly different results as compared to those are obtained from conventional methods used in quantum mechanics.
Acknowledgements
Authors would like to thank Dr. S.M. Desai for a careful reading of this article and for many helpful suggestions that have improved the content and clarity of presentation.
References